STATE EDUCATIONAL INSTITUTION

"Krivlyanskaya Secondary School"

ZHABINKOVSKY DISTRICT

FIBONACCI NUMBERS AND THE GOLDEN RATIO

Research

Work completed:

10th grade student

Sadovnichik Valeria Alekseevna

Supervisor:

Lavrenyuk Larisa Nikolaevna,

computer science teacher and

Mathematics 1 qualification

Fibonacci numbers and nature

A characteristic feature of the structure of plants and their development is spirality. Even Goethe, who was not only a great poet, but also a natural scientist, considered spirality to be one of the characteristic features of all organisms, a manifestation of the innermost essence of life. The tendrils of plants twist in a spiral, tissue growth in tree trunks occurs in a spiral, seeds in a sunflower are located in a spiral, spiral movements (nutations) are observed during the growth of roots and shoots.

At first glance, it may seem that the number of leaves and flowers can vary within very wide limits and take on any value. But such a conclusion turns out to be untenable. Research has shown that the number of organs of the same name in plants is not arbitrary; there are values ​​that are often found and values ​​that are very rare.

In living nature, forms based on pentagonal symmetry are widespread - starfish, sea urchins, flowers.

Photo 13. Buttercup

Chamomile has 55 or 89 petals.

Photo 14. Chamomile

Pyrethrum has 34 petals.

Phot. 15. Pyrethrum

Let's look at a pine cone. The scales on its surface are arranged strictly regularly - along two spirals that intersect approximately at a right angle. The number of such spirals in pine cones is 8 and 13 or 13 and 21.

Photo 16. Cone

In sunflower baskets, the seeds are also arranged in two spirals, their number is usually 34/55, 55/89.

Photo 17. Sunflower

Let's take a closer look at the shells. If you count the number of “stiffening ribs” of the first shell, taken at random, it turns out to be 21. Let’s take the second, third, fifth, tenth shell - they will all have 21 ribs on the surface. Apparently, the mollusks were not only good engineers, they “knew” Fibonacci numbers.

Photo 18. Shell

Here again we see a natural combination of Fibonacci numbers located nearby: 2/3, 3/5, 5/8, 8/13, 13/21, 21/34, 34/55, 55/89. Their ratio in the limit tends to the golden proportion, expressed by the number 0.61803...

Fibonacci numbers and animals

The number of rays of starfish corresponds to the Fibonacci numbers or is very close to them and is equal to 5.8, 13,21,34,55.

Photo 19. Starfish

Modern arthropods are very diverse. The lobster also has five pairs of legs, five feathers on the tail, the abdomen is divided into five segments, and each leg consists of five parts.

Phot. 20. lobster

In some insects, the abdomen consists of eight segments, there are three pairs of limbs consisting of eight parts, and eight different antennae-like organs emerge from the mouth opening. Our well-known mosquito has three pairs of legs, the abdomen is divided into eight segments, and there are five antennae on the head. The mosquito larva is divided into 12 segments.

Phot. 21. Mosquito

The cabbage fly's abdomen is divided into five parts, there are three pairs of legs, and the larva is divided into eight segments. Each of the two wings is divided into eight parts by thin veins.

The caterpillars of many insects are divided into 13 segments, for example, those of the skin beetle, mucous beetle, and Moorish booger. In most pest beetles, the caterpillar is divided into 13 segments. The structure of the legs of beetles is very characteristic. Each leg consists of three parts, as in higher animals - the shoulder, forearm and paw. The thin, openwork legs of beetles are divided into five parts.

The openwork, transparent, weightless wings of a dragonfly are a masterpiece of nature’s “engineering” mastery. What proportions are the basis for the design of this tiny flying muscle plane? The ratio of wingspan to body length in many dragonflies is 4/3. The dragonfly's body is divided into two main parts: a massive body and a long, thin tail. The body has three parts: head, chest, abdomen. The abdomen is divided into five segments, and the tail consists of eight parts. Here you also need to add three pairs of legs with their division into three parts.

Phot. 22. Dragonfly

It is not difficult to see in this sequence of dividing the whole into parts the unfolding of a series of Fibonacci numbers. The length of the tail, body and total length of a dragonfly are related to each other by the golden ratio: the ratio of the lengths of the tail and body is equal to the ratio of the total length to the length of the tail.

It is not surprising that the dragonfly looks so perfect, because it was created according to the laws of the golden ratio.

The sight of a turtle against the background of takyr covered with cracks is an amazing phenomenon. In the center of the shell there is a large oval field with large fused horny plates, and along the edges there is a border of smaller plates.

Phot. 23. Turtle

Take any turtle - from the close to us marsh turtle to the giant sea turtle - and you will be convinced that the pattern on their shell is similar: on the oval field there are 13 fused horny plates - 5 plates in the center and 8 at the edges, and on the peripheral border about 21 plates (the Chilean tortoise has exactly 21 plates along the periphery of its shell). Turtles have 5 toes on their feet, and the spinal column consists of 34 vertebrae. It is easy to see that all of these values ​​correspond to Fibonacci numbers. Consequently, the development of the turtle, the formation of its body, the division of the whole into parts was carried out according to the law of the Fibonacci number series.

The highest type of animals on the planet are mammals. The number of ribs in many animal species is equal to or close to thirteen. In completely different mammals - whale, camel, deer, aurochs - the number of ribs is 13 ± 1. The number of vertebrae varies greatly, especially due to the tails, which can be of different lengths even in the same species of animal. But in many of them the number of vertebrae is equal to or close to 34 and 55. So, a giant deer has 34 vertebrae, a whale has 55.

The skeleton of the limbs of domestic animals consists of three identical bone links: the humerus (pelvic) bone, the forearm bone (tibia) and the paw bone (foot). The foot, in turn, consists of three bone links.

The number of teeth in many domestic animals tends to Fibonacci numbers: a rabbit has 14 pairs, a dog, pig, and horse have 21 ± 1 pair of teeth. In wild animals, the number of teeth varies more widely: in one marsupial predator it is 54, in a hyena - 34, in one species of dolphin it reaches 233. The total number of bones in the skeleton of domestic animals (including teeth) in one group is close to 230, and in another - to 300. It should be noted that the number of bones of the skeleton does not include small auditory ossicles and unstable ossicles. Taking them into account, the total number of skeletal bones in many animals will be close to 233, and in others it will exceed 300. As we can see, the division of the body, accompanied by the development of the skeleton, is characterized by a discrete change in the number of bones in various organs of animals, and these numbers correspond to Fibonacci numbers or very close to them, forming a row 3, 5, 8, 13, 21, 34, 55, 89, 144, 233. The size ratio of most chicken eggs is 4:3 (some 3/2), pumpkin seeds - 3:2 , watermelon seeds - 3/2. The ratio of the length of pine cones to their diameter turned out to be 2:1. The sizes of birch leaves on average are very close to, and acorns - 5:2.

It is believed that if it is necessary to divide a flower lawn into two parts (grass and flowers), then these stripes should not be made equal in width; it will be more beautiful if you take them in a ratio of 5: 8 or 8: 13, i.e. use a proportion called the “golden ratio”.

Fibonacci numbers and photography

When applied to photographic art, the golden ratio divides the frame into 9 unequal rectangles by two horizontal and two vertical lines. To make it easier for themselves to capture balanced images, photographers simplified the task a little and began dividing the frame into 9 equal rectangles in accordance with the Fibonacci numbers. Thus, the rule of the golden ratio was transformed into the rule of thirds, which refers to one of the principles of composition.

Phot. 24. Frame and golden ratio

In the viewfinders of modern digital cameras, the focus points are located at 2/8 positions or on imaginary lines dividing the frame according to the golden ratio.

Photo 25. Digital camera and focus points

Photo 26.

Photo 27. Photography and Focus Points

The rule of thirds applies to all subject compositions: whether you are shooting a landscape or a portrait, a still life or a reportage. Until your sense of harmony becomes acquired and unconscious, following the simple rule of thirds will allow you to take pictures that are expressive, harmonious, and balanced.

Photo 28. Photography and the ratio of heaven and earth 1 to 2.

The most successful example for demonstration is a landscape. The principle of composition is that the sky and land (or water surface) should have a 1:2 ratio. One third of the frame should be allocated to the sky, and two thirds to the land, or vice versa.

Photo 29. Photo of a flower twisting in a spiral

Fibonacci and space

The ratio of water and land on planet Earth is 62% and 38%.

The sizes of the Earth and the Moon are in the golden ratio.

Photo 30. Sizes of the Earth and Moon

The figure shows the relative sizes of the Earth and Moon to scale.

Let's draw the radius of the Earth. Let us draw a segment from the central point of the Earth to the central point of the Moon, the length of which will be equal to). Let's draw a line segment to connect the two given line segments to form a triangle. We get a golden triangle.

Saturn shows the golden ratio in several of its dimensions

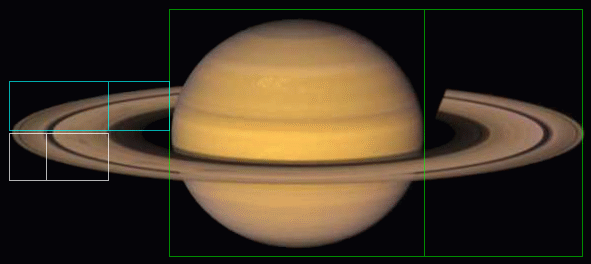
Photo 31. Saturn and its rings

Saturn's diameter is very closely related to the golden ratio with the diameter of the rings, as shown by the green lines.Radius inThe inner part of the rings is in a ratio very close to the outer diameter of the rings, as shown by the blue line.

The distance of planets from the Sun also follows the golden ratio.

Photo 32. Distance of planets from the Sun

Golden ratio in everyday life

The Golden Ratio is also used to impart style and appeal in the marketing and design of everyday consumer products. There are many examples, but we will illustrate only a few.

Photo 33. EmblemToyota

Photo 34. Golden ratio and clothing

Photo 34. The Golden Ratio and Automotive Design

Photo 35. EmblemApple

Photo 36. EmblemGoogle

Case studies

Now we will apply the acquired knowledge in practice. Let's first take measurements among 8th grade students.

If you look closely at a television picture, its dimensions will be 16 to 9 or 16 to 10, which is also close to the golden ratio.

Carrying out measurements and constructions in CorelDRAW X4 and using a frame from the Russia 24 news channel, you can find the following:

a) the ratio of the length to the width of the frame is 1.7.

b) the person in the frame is located exactly at the focus points located at a distance of 3/8.

Next, let's turn to the official microblog of the Izvestia newspaper, in other words, to the Twitter page. For a monitor screen with 4:3 sides, we see that the “header” of the page is 3/8 of the entire height of the page.

Taking a close look at the military caps, you can find the following:

a) the cap of the Minister of Defense of the Russian Federation has a ratio of the indicated parts of 21.73 to 15.52, equal to 1.4.

b) the cap of the border guard of the Republic of Belarus has the dimensions of the indicated parts 44.42 to 21.33, which is equal to 2.1.

c) the cap from the times of the USSR has the dimensions of the indicated parts 49.67 to 31.04, which is equal to 1.6.

For this model, the dress length is 113.13 mm.

If we “finish” the dress to the “ideal” length, we will get a picture like this.

All measurements have some error, since they were carried out from photographs, which does not interfere with seeing the trend - everything that is ideal contains the golden ratio to one degree or another.

Conclusion

The world of living nature appears to us completely different - mobile, changeable and surprisingly diverse. Life shows us a fantastic carnival of diversity and uniqueness of creative combinations! The world of inanimate nature is, first of all, a world of symmetry, which gives his creations stability and beauty. The natural world is, first of all, a world of harmony, in which the “law of the golden ratio” operates.

“The “golden ratio” seems to be that moment of truth, without which, in general, nothing existing is possible. Whatever we take as an element of research, the “golden ratio” will be everywhere; even if there is no visible observance of it, then it certainly takes place at the energetic, molecular or cellular levels.

Truly, nature turns out to be monotonous (and therefore unified!) in the manifestation of its fundamental laws. The “most successful” solutions she found apply to a wide variety of objects and to a wide variety of forms of organization. Continuity and discreteness of organization comes from the dual unity of matter - its corpuscular and wave nature, penetrates into chemistry, where it gives the laws of integer stoichiometry, chemical compounds of constant and variable composition. In botany, continuity and discreteness find their specific expression in phyllotaxis, quanta of discreteness, quanta of growth, the unity of discreteness and continuity of space-time organization. And now, in the numerical ratios of plant organs, the “principle of multiple ratios” introduced by A. Gursky appears - a complete repetition of the basic law of chemistry.

Of course, the statement that all these phenomena are based on the Fibonacci sequence sounds too loud, but the trend is obvious. And besides, she herself is far from perfect, like everything in this world.

There is an assumption that the Fibonacci series is an attempt by nature to adapt to a more fundamental and perfect golden ratio logarithmic sequence, which is almost the same, only it starts from nowhere and goes to nowhere. Nature definitely needs some kind of whole beginning from which it can start; it cannot create something out of nothing. The ratios of the first terms of the Fibonacci sequence are far from the Golden Ratio. But the further we move along it, the more these deviations are smoothed out. To define any series, it is enough to know its three terms, coming one after another. But not for the golden sequence, two are enough for it, it is a geometric and arithmetic progression at the same time. One might think that it is the basis for all other sequences.

Each term of the golden logarithmic sequence is a power of the Golden Proportion (). Part of the series looks something like this:... ; ; ; ; ; ; ; ; ; ; ... If we round the value of the Golden Ratio to three decimal places, we get=1,618 , then the series looks like this:... 0,090 0,146; 0,236; 0,382; 0,618; 1; 1,618; 2,618; 4,236; 6,854; 11,090 ... Each next term can be obtained not only by multiplying the previous one by1,618 , but also by adding the two previous ones. Thus, exponential growth is achieved by simply adding two adjacent elements. It's a series without beginning or end, and that's what the Fibonacci sequence tries to be like. Having a very definite beginning, she strives for the ideal, never achieving it. That is life.

And yet, in connection with everything we have seen and read, quite logical questions arise:
Where did these numbers come from? Who is this architect of the universe who tried to make it ideal? Was everything ever the way he wanted? And if so, why did it go wrong? Mutations? Free choice? What will be next? Is the spiral curling or unwinding?

Having found the answer to one question, you will get the next one. If you solve it, you'll get two new ones. Once you deal with them, three more will appear. Having solved them too, you will have five unsolved ones. Then eight, then thirteen, 21, 34, 55...

List of sources used

Vasyutinsky, N. Golden proportion / Vasyutinsky N, Moscow, Young Guard, 1990, - 238 p. - (Eureka).

Vorobyov, N.N. Fibonacci numbers,

Access mode: . Access date: 11/17/2015.

Access mode: . Access date: 16.11.2015.

Access mode: . Access date: 13.11.2015.

Let's find out what the ancient Egyptian pyramids, Leonardo da Vinci's Mona Lisa, a sunflower, a snail, a pine cone and human fingers have in common?

The answer to this question is hidden in the amazing numbers that have been discovered Italian medieval mathematician Leonardo of Pisa, better known by the name Fibonacci (born about 1170 - died after 1228), Italian mathematician . Traveling around the East, he became acquainted with the achievements of Arab mathematics; contributed to their transfer to the West.

After his discovery, these numbers began to be called after the famous mathematician. The amazing essence of the Fibonacci number sequence is that that each number in this sequence is obtained from the sum of the two previous numbers.

So, the numbers forming the sequence:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, 2584, …

are called “Fibonacci numbers”, and the sequence itself is called the Fibonacci sequence.

There is one very interesting feature about Fibonacci numbers. When dividing any number from the sequence by the number in front of it in the series, the result will always be a value that fluctuates around the irrational value 1.61803398875... and sometimes exceeds it, sometimes does not reach it. (Approx. irrational number, i.e. a number whose decimal representation is infinite and non-periodic)

Moreover, after the 13th number in the sequence, this division result becomes constant until the infinity of the series... It was this constant number of divisions that was called the Divine proportion in the Middle Ages, and is now called the golden ratio, the golden mean, or the golden proportion. . In algebra, this number is denoted by the Greek letter phi (Ф)

So, Golden ratio = 1:1.618

233 / 144 = 1,618

377 / 233 = 1,618

610 / 377 = 1,618

987 / 610 = 1,618

1597 / 987 = 1,618

2584 / 1597 = 1,618

The human body and the golden ratio

Artists, scientists, fashion designers, designers make their calculations, drawings or sketches based on the ratio of the golden ratio. They use measurements from the human body, which was also created according to the principle of the golden ratio. Before creating their masterpieces, Leonardo Da Vinci and Le Corbusier took the parameters of the human body, created according to the law of the Golden Proportion.

The most important book of all modern architects, E. Neufert’s reference book “Building Design,” contains basic calculations of the parameters of the human torso, which contain the golden proportion.

The proportions of the various parts of our body are a number very close to the golden ratio. If these proportions coincide with the golden ratio formula, then the person’s appearance or body is considered ideally proportioned. The principle of calculating the gold measure on the human body can be depicted in the form of a diagram:

M/m=1.618

The first example of the golden ratio in the structure of the human body:
If we take the navel point as the center of the human body, and the distance between a person’s foot and the navel point as a unit of measurement, then a person’s height is equivalent to the number 1.618.

In addition to this, there are several more basic golden proportions of our body:

* the distance from the fingertips to the wrist to the elbow is 1:1.618;

* the distance from shoulder level to the top of the head and the size of the head is 1:1.618;

* the distance from the navel point to the crown of the head and from shoulder level to the crown of the head is 1:1.618;

* the distance of the navel point to the knees and from the knees to the feet is 1:1.618;

* the distance from the tip of the chin to the tip of the upper lip and from the tip of the upper lip to the nostrils is 1:1.618;

* the distance from the tip of the chin to the upper line of the eyebrows and from the upper line of the eyebrows to the crown is 1:1.618;

* the distance from the tip of the chin to the top line of the eyebrows and from the top line of the eyebrows to the crown is 1:1.618:

The golden ratio in human facial features as a criterion of perfect beauty.

In the structure of human facial features there are also many examples that are close in value to the golden ratio formula. However, do not immediately rush for a ruler to measure the faces of all people. Because exact correspondences to the golden ratio, according to scientists and artists, artists and sculptors, exist only in people with perfect beauty. Actually, the exact presence of the golden proportion in a person’s face is the ideal of beauty for the human gaze.

For example, if we sum up the width of the two front upper teeth and divide this sum by the height of the teeth, then, having obtained the golden ratio number, we can say that the structure of these teeth is ideal.

There are other embodiments of the golden ratio rule on the human face. Here are a few of these relationships:

*Face height/face width;

* Central point of connection of the lips to the base of the nose / length of the nose;

* Face height / distance from the tip of the chin to the central point where the lips meet;

*Mouth width/nose width;

* Nose width / distance between nostrils;

* Distance between pupils / distance between eyebrows.

Human hand

It is enough just to bring your palm closer to you and look carefully at your index finger, and you will immediately find the formula of the golden ratio in it. Each finger of our hand consists of three phalanges.

* The sum of the first two phalanges of the finger in relation to the entire length of the finger gives the number of the golden ratio (with the exception of the thumb);

* In addition, the ratio between the middle finger and little finger is also equal to the golden ratio;

* A person has 2 hands, the fingers on each hand consist of 3 phalanges (except for the thumb). There are 5 fingers on each hand, that is, 10 in total, but with the exception of two two-phalanx thumbs, only 8 fingers are created according to the principle of the golden ratio. Whereas all these numbers 2, 3, 5 and 8 are the numbers of the Fibonacci sequence:

The golden ratio in the structure of the human lungs

American physicist B.D. West and Dr. A.L. Goldberger, during physical and anatomical studies, established that the golden ratio also exists in the structure of the human lungs.

The peculiarity of the bronchi that make up the human lungs lies in their asymmetry. The bronchi consist of two main airways, one of which (the left) is longer and the other (the right) is shorter.

* It was found that this asymmetry continues in the branches of the bronchi, in all the smaller airways. Moreover, the ratio of the lengths of short and long bronchi is also the golden ratio and is equal to 1:1.618.

Structure of the golden orthogonal quadrilateral and spiral

The golden ratio is such a proportional division of a segment into unequal parts, in which the entire segment is related to the larger part as the larger part itself is related to the smaller one; or in other words, the smaller segment is to the larger as the larger is to the whole.

In geometry, a rectangle with this aspect ratio came to be called the golden rectangle. Its long sides are in relation to its short sides in a ratio of 1.168:1.

The golden rectangle also has many amazing properties. The golden rectangle has many unusual properties. By cutting a square from the golden rectangle, the side of which is equal to the smaller side of the rectangle, we again obtain a golden rectangle of smaller dimensions. This process can be continued indefinitely. As we continue to cut off squares, we will end up with smaller and smaller golden rectangles. Moreover, they will be located in a logarithmic spiral, which is important in mathematical models of natural objects (for example, snail shells).

The pole of the spiral lies at the intersection of the diagonals of the initial rectangle and the first vertical one to be cut. Moreover, the diagonals of all subsequent decreasing golden rectangles lie on these diagonals. Of course, there is also the golden triangle.

English designer and esthetician William Charlton stated that people find spiral shapes pleasing to the eye and have been using them for thousands of years, explaining it this way:

“We like the look of a spiral because visually we can easily look at it.”

In nature

* The rule of the golden ratio, which underlies the structure of the spiral, is found in nature very often in creations of unparalleled beauty. The most obvious examples are that the spiral shape can be seen in the arrangement of sunflower seeds, pine cones, pineapples, cacti, the structure of rose petals, etc.;

* Botanists have found that in the arrangement of leaves on a branch, sunflower seeds or pine cones, the Fibonacci series is clearly manifested, and therefore the law of the golden ratio is manifested;

The Almighty Lord established a special measure for each of His creations and gave it proportionality, which is confirmed by examples found in nature. One can give a great many examples when the growth process of living organisms occurs in strict accordance with the shape of a logarithmic spiral.

All springs in the spiral have the same shape. Mathematicians have found that even with an increase in the size of the springs, the shape of the spiral remains unchanged. There is no other form in mathematics that has the same unique properties as the spiral.

The structure of sea shells

Scientists who studied the internal and external structure of the shells of soft-bodied mollusks living at the bottom of the seas stated:

“The inner surface of the shells is impeccably smooth, while the outer surface is completely covered with roughness and irregularities. The mollusk was in a shell and for this the inner surface of the shell had to be perfectly smooth. External corners-bends of the shell increase its strength, hardness and thus increase its strength. The perfection and amazing intelligence of the structure of the shell (snail) is amazing. The spiral idea of ​​shells is a perfect geometric form and is amazing in its honed beauty."

In most snails that have shells, the shell grows in the shape of a logarithmic spiral. However, there is no doubt that these unreasonable creatures not only have no idea about the logarithmic spiral, but do not even have the simplest mathematical knowledge to create a spiral-shaped shell for themselves.

But then how were these unreasonable creatures able to determine and choose for themselves the ideal form of growth and existence in the form of a spiral shell? Could these living creatures, which the scientific world calls primitive life forms, calculate that the logarithmic shell shape would be ideal for their existence?

Of course not, because such a plan cannot be realized without intelligence and knowledge. But neither primitive mollusks nor unconscious nature possess such intelligence, which, however, some scientists call the creator of life on earth (?!)

Trying to explain the origin of such even the most primitive form of life by a random combination of certain natural circumstances is absurd, to say the least. It is clear that this project is a conscious creation.

Biologist Sir D'arky Thompson calls this type of growth of sea shells "growth form of dwarves."

Sir Thompson makes this comment:

“There is no simpler system than the growth of sea shells, which grow and expand in proportion, maintaining the same shape. The most amazing thing is that the shell grows, but never changes shape.”

The Nautilus, measuring several centimeters in diameter, is the most striking example of the gnome growth habit. S. Morrison describes this process of nautilus growth as follows, which seems quite difficult to plan even with the human mind:

“Inside the nautilus shell there are many compartments-rooms with partitions made of mother-of-pearl, and the shell itself inside is a spiral expanding from the center. As the nautilus grows, another room grows in the front part of the shell, but this time it is larger than the previous one, and the partitions of the room left behind are covered with a layer of mother-of-pearl. Thus, the spiral expands proportionally all the time.”

Here are just some types of spiral shells with a logarithmic growth pattern in accordance with their scientific names:
Haliotis Parvus, Dolium Perdix, Murex, Fusus Antiquus, Scalari Pretiosa, Solarium Trochleare.

All discovered fossil remains of shells also had a developed spiral shape.

However, the logarithmic growth form is found in the animal world not only in mollusks. The horns of antelopes, wild goats, rams and other similar animals also develop in the form of a spiral according to the laws of the golden ratio.

Golden ratio in the human ear

In the human inner ear there is an organ called Cochlea (“Snail”), which performs the function of transmitting sound vibration. This bony structure is filled with fluid and is also shaped like a snail, containing a stable logarithmic spiral shape = 73º 43'.

Animal horns and tusks developing in a spiral shape

The tusks of elephants and extinct mammoths, the claws of lions and the beaks of parrots are logarithmic in shape and resemble the shape of an axis that tends to turn into a spiral. Spiders always weave their webs in the form of a logarithmic spiral. The structure of microorganisms such as plankton (species globigerinae, planorbis, vortex, terebra, turitellae and trochida) also have a spiral shape.

Golden ratio in the structure of microcosms

Geometric shapes are not limited to just a triangle, square, pentagon or hexagon. If we connect these figures with each other in different ways, we get new three-dimensional geometric figures. Examples of this are figures such as a cube or a pyramid. However, besides them, there are also other three-dimensional figures that we have not encountered in everyday life, and whose names we hear, perhaps for the first time. Among such three-dimensional figures are the tetrahedron (regular four-sided figure), octahedron, dodecahedron, icosahedron, etc. The dodecahedron consists of 13 pentagons, the icosahedron of 20 triangles. Mathematicians note that these figures are mathematically very easily transformed, and their transformation occurs in accordance with the formula of the logarithmic spiral of the golden ratio.

In the microcosm, three-dimensional logarithmic forms built according to golden proportions are ubiquitous . For example, many viruses have the three-dimensional geometric shape of an icosahedron. Perhaps the most famous of these viruses is the Adeno virus. The protein shell of the Adeno virus is formed from 252 units of protein cells arranged in a specific sequence. At each corner of the icosahedron there are 12 units of protein cells in the shape of a pentagonal prism and spike-like structures extend from these corners.

The golden ratio in the structure of viruses was first discovered in the 1950s. scientists from Birkbeck College London A. Klug and D. Kaspar. 13 The Polyo virus was the first to display a logarithmic form. The form of this virus turned out to be similar to the form of the Rhino 14 virus.

The question arises, how do viruses form such complex three-dimensional shapes, the structure of which contains the golden ratio, which are quite difficult to construct even with our human mind? The discoverer of these forms of viruses, virologist A. Klug, gives the following comment:

“Dr. Kaspar and I showed that for the spherical shell of the virus, the most optimal shape is symmetry such as the icosahedron shape. This order minimizes the number of connecting elements... Most of Buckminster Fuller's geodesic hemispherical cubes are built on a similar geometric principle. 14 Installation of such cubes requires an extremely accurate and detailed explanatory diagram. Whereas unconscious viruses themselves construct such a complex shell from elastic, flexible protein cellular units.”

based on materials from the book by B. Biggs “A hedger emerged from the fog”

About Fibonacci numbers and trading

As an introduction to the topic, let's briefly turn to technical analysis. In short, technical analysis aims to predict the future price movement of an asset based on past historical data. The most famous formulation of its supporters is that the price already includes all the necessary information. The implementation of technical analysis began with the development of stock market speculation and is probably not completely finished yet, since it potentially promises unlimited earnings. The most well-known methods (terms) in technical analysis are support and resistance levels, Japanese candlesticks, figures foreshadowing a price reversal, etc.

The paradox of the situation, in my opinion, is the following - most of the described methods have become so widespread that, despite the lack of evidence base on their effectiveness, they actually have the opportunity to influence market behavior. Therefore, even skeptics who use fundamental data should take these concepts into account simply because so many other players (“techies”) take them into account. Technical analysis can work well on history, but in practice almost no one manages to make stable money with its help - it is much easier to get rich by publishing a book in large quantities on “how to become a millionaire using technical analysis”...

In this sense, the Fibonacci theory stands apart, which is also used to predict prices for different periods. Her followers are usually called "wavers." It stands apart because it did not appear simultaneously with the market, but much earlier - as much as 800 years. Another feature of it is that the theory is reflected almost as a world concept for describing everything and everyone, and the market is only a special case for its application. The effectiveness of the theory and the period of its existence provide it with both new supporters and new attempts to create the least controversial and generally accepted description of the behavior of markets on its basis. But alas, the theory has not advanced beyond individual successful market predictions, which can be equated to luck.

The essence of Fibonacci theory

Fibonacci lived a long life, especially for his time, which he devoted to solving a number of mathematical problems, formulating them in his voluminous work “The Book of Abacus” (early 13th century). He was always interested in the mysticism of numbers - he was probably no less brilliant than Archimedes or Euclid. Problems related to quadratic equations were posed and partially solved before Fibonacci, for example by the famous Omar Khayyam, a scientist and poet; however, Fibonacci formulated the problem of the reproduction of rabbits, the conclusions from which brought him something that allowed his name not to be lost in the centuries.

Briefly, the task is as follows. A pair of rabbits was placed in a place fenced on all sides by a wall, and any pair of rabbits gives birth to another pair every month, starting from the second month of their existence. The reproduction of rabbits over time will be described by the sequence: 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, etc. From a mathematical point of view, the sequence turned out to be simply unique, since it had a number of outstanding properties:

• the sum of any two consecutive numbers is the next number in the sequence;

• the ratio of each number in the sequence, starting from the fifth, to the previous one is 1.618;

• the difference between the square of any number and the square of a number two positions to the left will be the Fibonacci number;

• the sum of the squares of adjacent numbers will be the Fibonacci number, which is two positions after the largest of the squared numbers

Of these findings, the second is the most interesting because it uses the number 1.618, known as the “golden ratio.” This number was known to the ancient Greeks, who used it during the construction of the Parthenon (by the way, according to some sources, the Central Bank served the Greeks). No less interesting is that the number 1.618 can be found in nature on both micro and macro scales - from the spiral turns on a snail’s shell to the large spirals of cosmic galaxies. The pyramids at Giza, created by the ancient Egyptians, also contained several parameters of the Fibonacci series during construction. A rectangle, one side of which is 1.618 times larger than the other, looks most pleasing to the eye - this ratio was used by Leonardo da Vinci for his paintings, and in a more everyday sense it was sometimes used when creating windows or doorways. Even a wave, as in the figure at the beginning of the article, can be represented as a Fibonacci spiral.

In living nature, the Fibonacci sequence appears no less often - it can be found in claws, teeth, sunflowers, spider webs and even the growth of bacteria. If desired, consistency is found in almost everything, including the human face and body. And yet, it is believed that many of the claims that find Fibonacci numbers in natural and historical phenomena are incorrect - this is a common myth that often turns out to be an inaccurate fit to the desired result.

Fibonacci numbers in financial markets

One of the first who was most closely involved in the application of Fibonacci numbers to the financial market was R. Elliot. His work was not in vain in the sense that market descriptions using Fibonacci theory are often called “Elliott waves.” The development of markets here was based on the model of human development from supercycles with three steps forward and two steps back. The fact that humanity is developing nonlinearly is obvious to almost everyone - the knowledge of Ancient Egypt and the atomistic teaching of Democritus was completely lost in the Middle Ages, i.e. after about 2000 years; The 20th century gave rise to such horror and insignificance of human life that it was difficult to imagine even in the era of the Punic Wars of the Greeks. However, even if we accept the theory of steps and their number as truth, the size of each step remains unclear, which makes Elliott waves comparable to the predictive power of heads and tails. The starting point and the correct calculation of the number of waves were and apparently will be the main weakness of the theory.

Nevertheless, the theory had local successes. Bob Pretcher, who can be considered a student of Elliott, correctly predicted the bull market of the early 1980s and saw 1987 as the turning point. This actually happened, after which Bob obviously felt like a genius - at least in the eyes of others, he certainly became an investment guru. Prechter's Elliott Wave Theorist subscription grew to 20,000 that year.however, it decreased in the early 1990s, as the further predicted "doom and gloom" of the American market decided to hold off a little. However, it worked for the Japanese market, and a number of supporters of the theory, who were “late” there for one wave, lost either their capital or the capital of their companies’ clients. In the same way and with the same success, they often try to apply the theory to trading in the foreign exchange market.

The theory covers a variety of trading periods - from weekly, which makes it similar to standard technical analysis strategies, to calculations for decades, i.e. gets into the territory of fundamental predictions. This is possible by varying the number of waves. The weaknesses of the theory, which were mentioned above, allow its adherents to speak not about the inconsistency of the waves, but about their own miscalculations among them and an incorrect definition of the starting position. It's like a labyrinth - even if you have the right map, you can only follow it if you understand exactly where you are. Otherwise the card is of no use. In the case of Elliott waves, there is every sign of doubting not only the correctness of your location, but also the accuracy of the map as such.

conclusions

The wave development of humanity has a real basis - in the Middle Ages, waves of inflation and deflation alternated with each other, when wars gave way to a relatively calm peaceful life. The observation of the Fibonacci sequence in nature, at least in some cases, also does not raise doubts. Therefore, everyone has the right to give their own answer to the question of who God is: a mathematician or a random number generator. My personal opinion is that although all of human history and markets can be represented in the wave concept, the height and duration of each wave cannot be predicted by anyone.

At the same time, 200 years of observing the American market and more than 100 years of other markets make it clear that the stock market is growing, going through various periods of growth and stagnation. This fact is quite enough for long-term earnings in the stock market, without resorting to controversial theories and trusting them with more capital than should be within reasonable risks.

Recently, working in individual and group processes with people, I have returned to thoughts about combining all processes (karmic, mental, physiological, spiritual, transformational, etc.) into one.

Friends behind the veil increasingly revealed the image of a multidimensional Man and the interconnection of everything in everything.

An inner urge prompted me to return to old studies with numbers and once again look through Drunvalo Melchizedek's book "The Ancient Secret of the Flower of Life."

At this time, the film "The Da Vinci Code" was shown in cinemas. It is not my intention to discuss the quality, value or truth of this film. But the moment with the code, when the numbers began to scroll rapidly, became one of the key moments in this film for me.

My intuition told me that it was worth paying attention to the Fibonacci number sequence and the Golden Ratio. If you look on the Internet to find something about Fibonacci, you will be bombarded with information. You will learn that this sequence has been known at all times. It is represented in nature and space, in technology and science, in architecture and painting, in music and proportions in the human body, in DNA and RNA. Many researchers of this sequence have come to the conclusion that key events in the life of a person, state, and civilization are also subject to the law of the golden ratio.

It seems that Man has been given a fundamental hint.

Then the thought arises that a Person can consciously apply the principle of the Golden Section to restore health and correct destiny, i.e. streamlining ongoing processes in one’s own universe, expanding Consciousness, returning to Well-Being.

Let's remember the Fibonacci sequence together:

1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, 2584, 4181, 6765, 10946, 17711, 28657, 46368, 75025…

Each subsequent number is formed by adding the two previous ones:

1+1=2, 1+2=3, 2+3=5, etc.

Now I propose to reduce each number in the series to one digit: 1, 1, 2, 3, 5, 8,

13=1+3(4), 21=2+1(3), 34=3+4(7), 55=5+5(1), 89= 8+9(8), 144=1+4+4(9)…

Here's what we got:

1, 1, 2, 3, 5, 8, 4, 3, 7, 1, 8, 9, 8, 8, 7, 6, 4, 1, 5, 6, 2, 8, 1, 9…1, 1, 2…

a sequence of 24 numbers that repeats again from the 25th:

75025=7+5+0+2+5=19=1+0=1, 121393=1+2+1+3+9+3=19=1+0=1…

Doesn't it seem strange or natural to you that

• there are 24 hours in a day,
• space houses - 24,
• DNA strands - 24,
• 24 elders from the God-Star Sirius,
• The repeating sequence in the Fibonacci series is 24 digits.

If the resulting sequence is written as follows,

1, 1, 2, 3, 5, 8, 4, 3, 7, 1, 8, 9

8, 8, 7, 6, 4, 1, 5, 6, 2, 8, 1, 9

9, 9, 9, 9, 9, 9, 9, 9, 9, 9, 9, 9,

then we will see that the 1st and 13th number of the sequence, the 2nd and 14th, the 3rd and 15th, the 4th and 16th... the 12th and 24th add up to 9 .

3 3 6 9 6 6 3 9

When testing these number series, we got:

• Child Principle;
• Fatherly Principle;
• Mother Principle;
• Principle of Unity.

Golden Ratio Matrix

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9

2 2 4 6 1 7 8 6 5 2 7 9 7 7 5 3 8 2 1 3 4 7 2 9

4 4 8 3 2 5 7 3 1 4 5 9 5 5 1 6 7 4 2 6 8 5 4 9

3 3 6 9 6 6 3 9 3 3 6 9 6 6 3 9 3 3 6 9 6 6 3 9

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9

8 8 7 6 4 1 5 6 2 8 1 9 1 1 2 3 5 8 4 3 7 1 8 9

8 8 7 6 4 1 5 6 2 8 1 9 1 1 2 3 5 8 4 3 7 1 8 9

8 8 7 6 4 1 5 6 2 8 1 9 1 1 2 3 5 8 4 3 7 1 8 9

7 7 5 3 8 2 1 3 4 7 2 9 2 2 4 6 1 7 8 6 5 2 7 9

4 4 8 3 2 5 7 3 1 4 5 9 5 5 1 6 7 4 2 6 8 5 4 9

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9

5 5 1 6 7 4 2 6 8 5 4 9 4 4 8 3 2 5 7 3 1 4 5 9

6 6 3 9 3 3 6 9 6 6 3 9 3 3 6 9 6 6 3 9 3 3 6 9

2 2 4 6 1 7 8 6 5 2 7 9 7 7 5 3 8 2 1 3 4 7 2 9

8 8 7 6 4 1 5 6 2 8 1 9 1 1 2 3 5 8 4 3 7 1 8 9

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9

9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9 9

Practical application of the Fibonacci series

One of my friends expressed his intention to work individually with him on the topic of developing his capabilities and abilities.

Unexpectedly, at the very beginning, Sai Baba came into the process and invited me to follow him.

We began to rise up inside the Divine Monad of our friend and, leaving it through the Causal Body, we found ourselves in another reality at the level of the Cosmic House.

Those who have studied the works of Mark and Elizabeth Claire Prophets know the teaching about the Cosmic Clock that Mother Mary conveyed to them.

At the level of the Cosmic House, Yuri saw a circle with an inner center with 12 arrows.

The elder who met us at this level said that before us the Divine Clock and 12 hands represent 12 (24) Manifestations of Divine Aspects... (possibly Creators).

As for the Cosmic Clock, they were located under the Divine Clock according to the principle of the energy eight.

— In what mode are the Divine Clocks in relation to you?

— The clock hands are standing still, there is no movement.Now thoughts are coming to me that many eons ago I abandoned the Divine Consciousness and followed a different path, the path of the Magician. All my magical artifacts and amulets, which I have and have accumulated in me over many incarnations, at this level look like baby rattles. On the subtle plane, they represent an image of magical energy clothing.

— Completed.However, I bless my magical experience.Living this experience truly motivated me to return to the source, to wholeness.They offer me to take off my magical artifacts and stand in the center of the Clock.

— What needs to be done to activate the Divine Clock?

— Sai Baba appeared again and offers to express the intention to connect the Silver String with the Clock. He also says that you have some kind of number series. He is the key to activation. The image of Leonard da Vinci's Man appears before your mind's eye.

- 12 times.

“I ask you to God-center the entire process and direct the energy of the number series to activate the Divine Clock.

Read aloud 12 times

1 1 2 3 5 8 4 3 7 1 8 9 8 8 7 6 4 1 5 6 2 8 1 9…

In the process of reading, the hands on the Clock moved.

Energy flowed along the silver string, connecting all levels of Yurina’s Monad, as well as earthly and heavenly energies...

The most unexpected thing in this process was that four Entities appeared on the Clock, which are some parts of the One Whole with Yura.

During communication, it became clear that once there was a division of the Central Soul, and each part chose its own area in the universe for implementation.

The decision was made to integrate, which happened at the Divine Hours center.

The result of this process was the creation of the Common Crystal at this level.

After this, I remembered that Sai Baba once spoke about a certain Plan, which involves first connecting two Essences into one, then four, and so on according to the binary principle.

Of course, this number series is not a panacea. This is just a tool that allows you to quickly carry out the necessary work with a person, to align him vertically with different levels of Being.

The Italian mathematician Leonardo Fibonacci lived in the 13th century and was one of the first in Europe to use Arabic (Indian) numerals. He came up with a somewhat artificial problem about rabbits being raised on a farm, all of which are considered females and the males are ignored. Rabbits begin breeding after they are two months old and then give birth to a rabbit every month. Rabbits never die.

We need to determine how many rabbits will be on the farm in n months, if at the initial time there was only one newborn rabbit.

Obviously, the farmer has one rabbit in the first month and one rabbit in the second month. By the third month there will be two rabbits, by the fourth month there will be three, etc. Let us denote the number of rabbits in n month like . Thus,
,
,
,
,
, …

It is possible to construct an algorithm to find at any n.

According to the problem statement, the total number of rabbits
V n+1 month is divided into three components:

one-month-old rabbits incapable of reproducing, in the amount of

;

Thus, we get

. (8.1)

Formula (8.1) allows you to calculate a series of numbers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, ...

The numbers in this sequence are called Fibonacci numbers .

If we accept
And
, then using formula (8.1) you can determine all other Fibonacci numbers. Formula (8.1) is called recurrent formula ( recurrence – “return” in Latin).

Example 8.1. Suppose there is a staircase in n steps. We can climb it in steps of one step, or in steps of two steps. How many combinations of different lifting methods are there?

If n= 1, there is only one solution to the problem. For n= 2 there are 2 options: two single steps or one double. For n= 3 there are 3 options: three single steps, or one single and one double, or one double and one single.

In the following case n= 4, we have 5 possibilities (1+1+1+1, 2+1+1, 1+2+1, 1+1+2, 2+2).

In order to answer the question asked at random n, let us denote the number of options as , and let's try to determine
according to known And
. If we start with a single step, then we have combinations for the remaining n steps. If we start with a double step, then we have
combinations for the remaining n–1 steps. Total number of options for n+1 steps equals

. (8.2)

The resulting formula resembles formula (8.1) as a twin. However, this does not allow us to identify the number of combinations with Fibonacci numbers . We see, for example, that
, But
. However, the following dependence takes place:

.

This is true for n= 1, 2, and also true for everyone n. Fibonacci numbers and number of combinations are calculated using the same formula, but the initial values
,
And
,
they differ.

Example 8.2. This example is of practical importance for problems of error-correcting coding. Find the number of all binary words of length n, not containing several zeros in a row. Let's denote this number by . Obviously,
, and words of length 2 that satisfy our constraint are: 10, 01, 11, i.e.
. Let
- such a word from n characters. If the symbol
, That
can be arbitrary (
)-literal word that does not contain several zeros in a row. This means that the number of words ending in one is
.

If the symbol
, then definitely
, and the first
symbol
may be arbitrary, subject to the constraints considered. Therefore, there is
words length n with a zero at the end. Thus, the total number of words we are interested in is equal to

.

Considering that
And
, the resulting sequence of numbers is the Fibonacci numbers.

Example 8.3. In Example 7.6 we found that the number of binary words of constant weight t(and length k) equals . Now let's find the number of binary words of constant weight t, not containing several zeros in a row.

You can think like this. Let
the number of zeros in the words in question. Any word has
spaces between nearest zeros, each of which contains one or more ones. It is assumed that
. Otherwise, there is not a single word without adjacent zeros.

If we remove exactly one unit from each interval, we get a word of length
containing zeros. Any such word can be obtained in the indicated way from some (and only one) k-literal word containing zeros, no two of which are adjacent. This means that the required number coincides with the number of all words of length
, containing exactly zeros, i.e. equals
.

Example 8.4. Let us prove that the sum
equal to Fibonacci numbers for any integer . Symbol
stands for smallest integer greater than or equal to . For example, if
, That
; and if
, That
ceil("ceiling"). There is also a symbol
, which denotes largest integer less than or equal to . In English this operation is called floor ("floor").

If
, That
. If
, That
. If
, That
.

Thus, for the cases considered, the sum is indeed equal to the Fibonacci numbers. Now we present the proof for the general case. Since the Fibonacci numbers can be obtained using the recurrence equation (8.1), the equality must be satisfied:

.

And it actually works:

Here we used the previously obtained formula (4.4):
.

Sum of Fibonacci numbers

Let us determine the sum of the first n Fibonacci numbers.

0+1+1+2+3+5 = 12,

0+1+1+2+3+5+8 = 20,

0+1+1+2+3+5+8+13 = 33.

It is easy to see that by adding one to the right side of each equation we again get the Fibonacci number. General formula for determining the sum of the first n Fibonacci numbers have the form:

Let's prove this using the method of mathematical induction. To do this, let's write:

This amount should be equal
.

Reducing the left and right sides of the equation by –1, we obtain equation (6.1).

Formula for Fibonacci numbers

Theorem 8.1. Fibonacci numbers can be calculated using the formula

.

Proof. Let us verify the validity of this formula for n= 0, 1, and then we will prove the validity of this formula for an arbitrary n by induction. Let's calculate the ratio of the two closest Fibonacci numbers:

We see that the ratio of these numbers fluctuates around 1.618 (if we ignore the first few values). This property of Fibonacci numbers resembles the terms of a geometric progression. Let's accept
, (
). Then the expression

converted to

which after simplifications looks like this

.

We have obtained a quadratic equation whose roots are equal:

Now we can write:

(Where c is a constant). Both members And do not give Fibonacci numbers, for example
, while
. However, the difference
satisfies the recurrence equation:

For n=0 this difference gives , that is:
. However, when n=1 we have
. To obtain
, you must accept:
.

Now we have two sequences: And
, which start with the same two numbers and satisfy the same recurrence formula. They must be equal:
. The theorem has been proven.

When increasing n member becomes very large while
, and the role of the member the difference is reduced. Therefore, at large n we can approximately write

.

We ignore 1/2 (since Fibonacci numbers increase to infinity as n to infinity).

Attitude
called golden ratio, it is used outside of mathematics (for example, in sculpture and architecture). The golden ratio is the ratio between the diagonal and the side regular pentagon(Fig. 8.1).

Rice. 8.1. Regular pentagon and its diagonals

To denote the golden ratio, it is customary to use the letter
in honor of the famous Athenian sculptor Phidias.

Prime numbers

All natural numbers, large ones, fall into two classes. The first includes numbers that have exactly two natural divisors, one and itself, the second includes all the rest. First class numbers are called simple, and the second – composite. Prime numbers within the first three tens: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, ...

The properties of prime numbers and their relationship with all natural numbers were studied by Euclid (3rd century BC). If you write down prime numbers in a row, you will notice that their relative density decreases. For the first ten there are 4, i.e. 40%, for a hundred – 25, i.e. 25%, per thousand – 168, i.e. less than 17%, per million – 78498, i.e. less than 8%, etc. However, their total number is infinite.

Among prime numbers there are pairs of such numbers, the difference between which is equal to two (the so-called simple twins), however, the finiteness or infinity of such pairs has not been proven.

Euclid considered it obvious that by multiplying only prime numbers one can obtain all natural numbers, and each natural number can be represented as a product of prime numbers in a unique way (up to the order of the factors). Thus, prime numbers form a multiplicative basis of the natural series.

The study of the distribution of prime numbers led to the creation of an algorithm that allows one to obtain tables of prime numbers. Such an algorithm is sieve of Eratosthenes(3rd century BC). This method consists of eliminating (for example, by striking out) those integers of a given sequence
, which are divisible by at least one of the prime numbers smaller
.

Theorem 8 . 2 . (Euclidean theorem). The number of prime numbers is infinite.

Proof. We will prove Euclid’s theorem on the infinity of the number of prime numbers using the method proposed by Leonhard Euler (1707–1783). Euler considered the product over all prime numbers p:

at
. This product converges, and if it is expanded, then, due to the uniqueness of the decomposition of natural numbers into prime factors, it turns out that it is equal to the sum of the series , from which Euler’s identity follows:

.

Since when
the series on the right diverges (harmonic series), then Euclid’s theorem follows from Euler’s identity.

Russian mathematician P.L. Chebyshev (1821–1894) derived a formula that determines the limits within which the number of prime numbers lies
, not exceeding X:

,

Where
,
.